
Education
Minimum transportation distance calculator
When doing a life cycle analysis of biodiesel, the average travel distance feedstock to get to the crushing plant matters. A longer hauling distance translates to more fuel consumption which translates to higher greenhouse gas emissions per unit of biodiesel made. A real-world transportation system’s efficiency can be evaluated by comparing the current hauling distance to an ideal minimum distance.
The calculator below can help determine theoretical minimum distance a feedstock must travel to a crushing facility for a given a crop yield, oil content, crop rotation, and crushing plant capacity.
What impacts the hauling distance?
There are several factors that impact the minimum hauling distance to supply feedstock to a crushing plant. We will consider them briefly in the following discussion:
1. Plant location
There are some assumptions to be made before we proceed. The first question is where the crushing plant is located relative to the agricultural land that grows the soybean? For a minimum distance calculator, it is obvious that the crushing plant would be located at the center of a field.
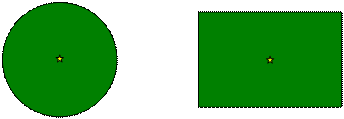
Figure 1: A crushing plant must be located at area center of the agricultural land to minimize the hauling distance. For, any shape of filed the distance to be hauled would be minimized if the plant is located at shape's area center.
2. Field shapes
Of the all possible field shapes, the hauling distance is minimum for a circular area. In larger geographical locations, however, there is going to be more than one crushing plants and the beans grown in between circles will have to go to one of the plants unless there is an additional plant in the middle of a gray area. However, if we imagine a plant in the middle of the gray area we would also have to rezone the area to minimize the hauling distance as shown in figure 2(b). Ultimately, a collection of square areas is needed to cover a larger landscape.
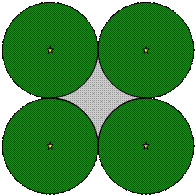 (a) |
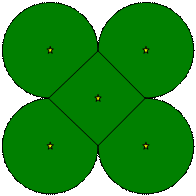 (b) |
Figure 2. (a) If all plants draw from the circular area, the soybean grown in gray zone is not covered. (b) If an additional plant is opened to cover the gray area, the rezoning will have to occur to minimize the hauling distance. Ultimately, it has got to be a collection of squares to cover all the larger agricultural land.
3. Crop rotation
Not all crops around an oil crushing plant are going to be oil crops. For example, in a corn-soybean rotation, if corn and soybean rotated every other year, there will be only 50% of the land under soybean. Since only soybean is being hauled to the crushing facility, it translates to hauling from twice as much area as is the soybean is planted all over. For a corn-corn-soybean rotation, the area around the plant feeding to the plant would be three times as much.
4. Crop Yield
For a given capacity of crushing plant, higher the average crop yield (more oil per acre) less is the amount of crop it must process.
5. Crushing plant size
Crushing plant size does matter. Bigger the crushing capacity larger will be the area that would satisfy plant demand and hence higher the average hauling distance.
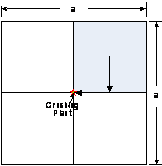
Figure 3. For a square filed of size a x a as shown above, assume that the crushing plant is located at the center. Let’s first consider the upper right ¼ ield (shaded corner). The average distance traveled from the shaded area to transport soybean to the crushing plant would be ‘ ½ a ’ (= ¼ a+ ¼ a). Now because of the symmetry, average distance travel from each of the 4 squares will also be ‘½ a’. Therefore, the average hauling distance from the entire field to its center would still be ‘½ a’. Hence, for a field of size a2, the minimum average hauling distance is ½ a.